AP Physics Lab
Hooke's Law


![[Help]](../../NavIcons/Help.GIF) [Lab Index]
[Lab Index]
BHS
-> Staff
-> Mr. Stanbrough ->
AP Physics-> Measurement->
this page
Purpose:
To investigate Hooke's Law.
Discussion:
Everybody knows that when you apply a force to a spring or a
rubber band, it stretches. A scientist would ask, "How is the force
that you apply related to the amount of stretch?" This question was
answered by Robert
Hooke, a contemporary and rival of Isaac
Newton, and the answer has come to be called Hooke's Law.
Hooke's Law says that the stretch of a spring from its rest
position is proportional to the applied force (or as the engineers
put it: stress is proportional to strain). Symbolically,
F = kx
where F stands for the applied force, x is the amount of stretch,
and k is a constant that depends on the "stiffness" of the spring,
often called the "spring constant".
Hooke's Law, believe it or not, is a very important and
widely-used law in physics and engineering. Its applications go far
beyond springs and rubber bands. The chair in which you are sitting
supplies the upward support force to keep you from falling by flexing
(according to Hooke's Law) until it can supply an upward support
force equal to your weight. The floor beneath your feet works the
same way.
You can investigate Hooke's Law by measuring how much known forces
stretch a spring. A convenient way to apply a precisely-known force
is to let the weight of a known mass be the force used to stretch the
spring. The force can be calculated from W = mg. The stretch of the
spring can be measured by noting the position of the end of the
spring before and during the application of the force.
Modern computer software takes just about all of the drudgery out
of data analysis. You can add calculated columns to a Graphical
AnalysisTM data table much as you would in a
spreadsheet. and drawing graphs is a snap - or rather, a click!.
Equipment:
|
small springs
|
rubber band
|
ring stand
|
|
|
ring-stand clamps
|
c-clamp
|
spring scale
|
ruler or meter stick
|
|
set of known masses
|
Graphical AnalysisTM software
|
|
|

Safety Notes:
- Be sure to keep your feet out of the area in
which the masses will fall if the spring or rubber band breaks!
- Be sure to clamp the ring stand to the lab table, or weight it
with several books so that the mass does not pull it off the
table.
- You need to hang enough mass to the end of the spring to get a
measurable stretch, but too much force will permanently
damage the spring. (An engineer would say that it has
exceeded its "elastic limit").
Setup:
- Assemble the apparatus as shown in the diagram at right. Be
sure to clamp the ring stand to the lab table, or weight it with
several books.
- Some springs tend to be "clenched" - their coils are pressing
against each other, and it takes a small force to simply get the
spring to the point that it will begin to stretch. If this is the
case, you may want to hang a small mass (20 g - 50 g) from the
spring initially and consider that to be the spring's starting
position.
- Spend a little mental effort considering how you are going to
measure the stretch of the spring precisely. Making a small
pointer out of a bent paper clip and fixing it to the end of the
spring has worked well for me in the past.
 The
data that you will need to record are the rest position of the
spring (same for each trial), stretched position of the spring,
and the total mass hanging from the spring. To set up a data table
(a sample is shown at right):
The
data that you will need to record are the rest position of the
spring (same for each trial), stretched position of the spring,
and the total mass hanging from the spring. To set up a data table
(a sample is shown at right):
- Open
the Graphical AnalysisTM program.
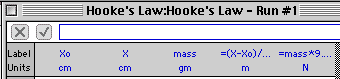
- Label the
first column to hold the rest position of the spring. I
used Xo in the example at right.
- Label the second column to hold the stretched
position.
- Add a
column to the data table for the hanging mass.
- Add a data column to hold the stretch of a spring (X - Xo).
In the example, I have converted this value to meters - the
actual formula is
"=(X-Xo)/100".
- Add a data column to hold the force used to stretch the
spring, which is the weight of the masses that you used. The
formula used in the sample spreadsheet is "=mass*9.81/1000",
which gives the force in Newtons.
- Graph force (horizontal
axis, since this is the quantity you change directly - the
independent variable) vs. stretch (vertical axis, since this is
the dependent variable)
- Change
the axes to display a force vs. stretch graph.
Procedure:
- For each trial, record the starting position of the spring
(before hanging the mass) and the ending position of the spring
(while it is being stretched), and the total mass. (For most of
our springs, starting with 50 gm and proceding in 50 gm increments
will be fine, but use some judgement and keep your eye on the
graph.)
- Add a
new data set (and a new graph) to Graphical
AnalysisTM, and repeat the process for another
spring, and a rubber band.
Results:
- You should be able to estimate
the uncertainty in measuring the rest and stretched positions
of the spring - and justify your estimate. You can then calculate
the uncertainty in the stretch
calculation. The manufacturer of the hooked masses we use
guarantee that they are within 2% of the "real mass", and we will
take their word for it. Add
error bars to your graph.
- If the data points look like a straight line, you can simply
add a best fit regression
line and regression
statistics to the graph - otherwise, you will need to try a
curve fit of
some type.
Conclusions & Questions:
- Do your results confirm or contradict Hooke's Law? Please
elaborate.
- What measurement contributed the most uncertainty to your
results? What could be done to improve it?
- What is the value of the spring constant, k, for each of your
springs? Show a sample calculation, please.
Questions:


![[Help]](../../NavIcons/Help.GIF) [Lab Index]
[Lab Index]
BHS
-> Staff
-> Mr. Stanbrough ->
AP Physics-> Measurement->
this page
last
update September 13, 2002 by JL
Stanbrough
 The
data that you will need to record are the rest position of the
spring (same for each trial), stretched position of the spring,
and the total mass hanging from the spring. To set up a data table
(a sample is shown at right):
The
data that you will need to record are the rest position of the
spring (same for each trial), stretched position of the spring,
and the total mass hanging from the spring. To set up a data table
(a sample is shown at right):
