Interpreting Absolute Value

Appendix A of the Stewart text (p. A6-A9) discusses absolute value in terms of distance, and everything that it says is true. What it doesn't tell you, however, is that if you interpret absolute value as distance you can solve most inequalities involving absolute value with a very simple number-line graph, and no algebra at all. Here is how it works.

How to Read an Absolute Value Statement:

If you read an absolute value statement correctly, the "battle" is almost over.

When you see "| x - a |" you should read it as "the distance from x to a".
So, the statement "|x - 2|" means "the distance from x to 2".
The statement "|x - 2| < 4" means "the distance from x to 2 is less than 4".
The statement "|x - 6| > 3" means "the distance from x to 6 is greater than 3".

"Ok, smarty", you might be thinking, "What does |x + 2| mean then? Well, |x + 2| = |x - (-2)|, right, so:

The statement "|x + 2| > 1" means "the distance from x to -2 is greater than 1".
The statement "|x +8| < 4" means "the distance from x to -8 is less than 4".

"What about |x|?" you ask? Well, |x| = |x - 0|, so:

The statement "|x| > 5" means "the distance from x to 0 is greater than 5".

Solving Inequalities Involving Absolute Value:

Example 1:

Let's solve the inequality "|x - 6| > 3", which is read "the distance from x to 6 is greater than 3".

Step 1: draw a number line and label "6".

Step 2: Find the points a distance 3 units away from 6.
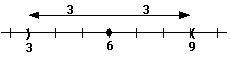step 2

Step 3: Since x must be farther than 3 units from 6, the shade the portions of the number line where x can be located. Here x < 3 or x > 9. This is the solution to the inequality.
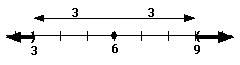step 3

Example 2:

Solve the inequality |x + 8| < 4. First, read this statement as "the distance from x to -8 is less than 4".

Step 1: Draw a number line and label "-8".

Step 2: Find the points a distance 4 units away from -8.
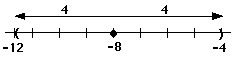step 2

Step 3: Since x must be closer than 4 units from -8, the shade the portion of the number line where x can be located. Here -12 < x < -4. This is the solution to the inequality.
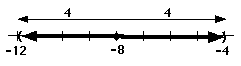step 3

Example 3:

Solve the inequality |3x - 6| < 9. First, read this statement as "the distance between 3x and 6 is less than 9".

Step 1: Draw a number line and label "6".
Step 2: Find the points a distance 9 units away from 6.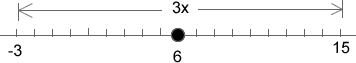
Step 3: From the diagram, -3 < 3x < 15
Step 4: Solve this simple inequality.	-1 < x < 5

last update August 22, 2007 by JL Stanbrough

Step 1: draw a number line and label "6".
Step 2: Find the points a distance 3 units away from 6.
Step 3: Since x must be farther than 3 units from 6, the shade the portions of the number line where x can be located. Here x < 3 or x > 9. This is the solution to the inequality.

Step 1: Draw a number line and label "-8".
Step 2: Find the points a distance 4 units away from -8.
Step 3: Since x must be closer than 4 units from -8, the shade the portion of the number line where x can be located. Here -12 < x < -4. This is the solution to the inequality.