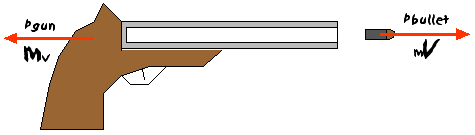
Before Firing:

Let's take our system as "gun plus bullet". This is an isolated system, since no outside forces exert impulses on the system. (We are ignoring gravity and air resistance.) The gun and the bullet are both at rest, so the momenta (mv) of the gun and the bullet are both zero. So, the total momentum of the system before the bullet is fired is zero.

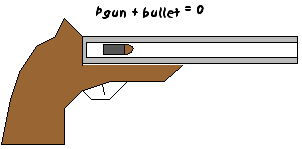
After Firing:

After the gun is fired, the bullet gets a velocity to the right, as shown. This means that the bullet now has momentum, mv, to the right. Since there was zero total momentum in the system before the gun was fired, there must be zero total momentum now. This means that the gun must have an equal and opposite momentum to the left, so:

p_gun = -p_bullet

Notice that even though the momenta of the gun and bullet are equal, their resulting velocities are not equal. The bullet has a small mass, so it gets a large velocity. The gun has a large mass, so it gets a small velocity. (The gun's velocity is the gun's "kick".)

M_gunv_gun = -m_bulletV_bullet