Falling, Sliding and Rolling

Introduction:

This page compares three interesting dynamical situations - free fall, sliding down a frictionless ramp, and rolling down a ramp. It is instructive to study the similarities and differences in these situations.

Free Fall:

Free-fall diagram Suppose you drop an object of mass m. If air resistance is not a factor in its fall (free fall), then the only force pulling on the object is its weight, mg. Therefore, the net force on the object equals its weight and Newton's Second Law says:

This result means that any object, regardless of its size or mass, will fall with the same acceleration (g = 9.8 m/s²) if air resistance can be ignored.

Sliding Down an Incline:

Next, let's consider letting objects slide down a frictionless ramp. Now, there are 2 forces on the object - its weight pulls down (toward the center of the Earth) and the ramp pushes upward, perpendicular to the surface of the ramp (the "normal" force). What happens is that, again, mass cancels out of Newton's Second Law, and the result is the prediction that all objects, regardless of mass or size, will slide down a frictionless incline at the same rate. The "gory details" are given in the table below, if you are interested. (For our purposes, you don't need to know the details.)

The two forces on the sliding object are its weight (= mg) pulling straight down (toward the center of the Earth) and the upward force that the ramp exerts (the "normal" force) perpendicular to the ramp.
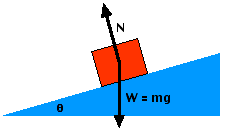Forces on an incline

Replacing the weight force by its components parallel and perpendicular to the incline, you can see that the weight component perpendicular to the incline cancels the normal force. This means that the net force equals the component of the weight parallel to the ramp, and Newton's 2^nd Law says:

This means that any object, regardless of size or mass, will slide down a frictionless ramp with the same acceleration (a fraction of g that depends on the angle of the ramp).
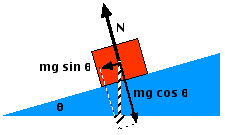sliding on an incline - with force components

Rolling Down an Incline:

Lastly, let's try rolling objects down an incline. This situation is more complicated, but more interesting, too.

First, recall that objects resist linear accelerations due to their mass - more mass means an object is more difficult to accelerate. However, objects resist rotational accelerations due to their rotational inertia (also called moment of inertia) - more rotational inertia means the object is more difficult to accelerate. Rotational inertia depends on:

the object's mass - more mass means more rotational inertia, and
how the object's mass is arranged relative to the axis of rotation - if the mass is farther from the axis, you get more rotational inertia.

Suppose that you have several round objects that have the same mass and radius, but made in different shapes. (This would be difficult in practice.) The objects below are listed with the greatest rotational inertia first:

a hoop - has the greatest rotational inertia, since all of its mass is as far as possible from its axis (center).
an empty can with no lid.
a hollow sphere - like a basketball, soccer ball, or volleyball.
a can of "sloshy" soup (chicken noodle) will have a higher rotational inertia than a "solid" can because of the "sloshing" of the contents.
a can of "solid" soup (a solid cylinder).
a solid sphere - like a billiard ball, has its mass packed as closely as possible to its axis of rotation, so it has the least rotational inertia.

If you "race" these objects down the incline, they would definitely not tie! This is because Newton's Second Law for Rotation says that the rotational acceleration of an object equals the net torque on the object divided by its rotational inertia. (Net torque replaces net force, and rotational inertia replaces mass in "regular" Newton's Second Law.) The net torque on every object would be the same - due to the weight of the object acting through its center of gravity, but the rotational inertias are different. This means that the solid sphere would beat the solid cylinder (since it has a smaller rotational inertia), the solid cylinder would beat the "sloshy" cylinder, etc. The hoop would come in last in every race, since it has the greatest moment of inertia (resistance to rotational acceleration).

Now, things get really interesting. What if you don't worry about matching each object's mass and radius? In other words, you find any old hoop, any hollow ball, any can of soup, etc., and race them. What happens then? The result is surprising!

It turns out, that if you calculate the rotational acceleration of a hoop, for instance, which equals (net torque)/(rotational inertia), both the torque and the rotational inertia depend on the mass and radius of the hoop. This means that both the mass and radius cancel in Newton's Second Law - just like what happened in the falling and sliding situations above! (The mathematical details are a little complex, but are shown in the table below) This means that all hoops, regardless of size or mass, roll at the same rate down the incline! The same is true for empty cans - all empty cans roll at the same rate, regardless of size or mass. However, every empty can will beat any hoop! All solid spheres roll with the same acceleration, but every solid sphere, regardless of size or mass, will beat any solid cylinder! Cool, huh?

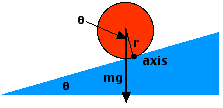
A circular object of mass m is rolling down a ramp that makes an angle with the horizontal. The weight, mg, of the object exerts a torque through the object's center of mass. The object rotates about its point of contact with the ramp, so the length of the lever arm equals the radius of the object.

Now, the component of the object's weight perpendicular to the radius is shown in the diagram at right. This means that the torque on the object about the contact point is given by:

and the rotational acceleration of the object is:

where I is the moment of inertia of the object. Now the moment of inertia of the object = kmr², where k is a constant that depends on how the mass is distributed in the object - k is different for cylinders and spheres, but is the same for all cylinders, and the same for all spheres. The rotational acceleration, then is:

So, the rotational acceleration of the object does not depend on its mass, but it does depend on its radius. However, we are really interested in the linear acceleration of the object down the ramp, and:

This result says that the linear acceleration of the object down the ramp does not depend on the object's radius or mass, but it does depend on how the mass is distributed. Therefore, all spheres have the same acceleration on the ramp, and all cylinders have the same acceleration on the ramp, but a sphere and a cylinder will have different accelerations, since their mass is distributed differently. All spheres "beat" all cylinders. All cylinders beat all hoops, etc.

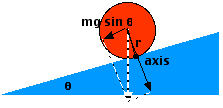last update April 21, 2008 by JL Stanbrough