Physics Lab

Galileo's Inclined Plane Experiment

(uses a Pasco Interface)

[Help] [Lab Index]

BHS -> Staff -> Mr. Stanbrough -> AP Physics-> Kinematics-> this page

Purpose:

To measure the acceleration of free fall, "g", using an inclined plane.

Discussion:

Inclined plane diagram

Around 1600, the amazing Galileo Galilei was able to show, experimentally, not only that the acceleration of objects in free fall was constant, but was able to determine a pretty good value for this acceleration. Galileo performed this feat by rolling wooden balls down long inclined planes, and he didn't even have the luxury of a clock!

Today, we can recreate Galileo's triumph with very little effort using an air track or dynamics track and a motion sensor.

Theory:

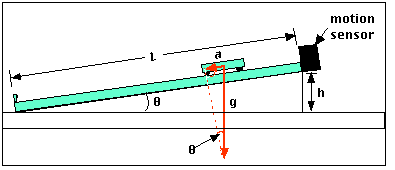
If a is the cart's acceleration down the track, and theta is the angle that the ramp makes with the horizontal, then from the diagram:

a = (g/L)h

so the graph of a vs. h should be a straight line with slope g/L.

Measuring h and L is pretty straightforward. You can determine the acceleration, a, from the slope of the velocity vs. time graph produced by the motion sensor for the motion. This isn't the way Galileo did it, but it's pretty slick!

Equipment:

Pasco Science WorkshopTM interface

motion sensor

meter stick/metric ruler

air track or dynamics track

ring stand & clamp (optional)

notebooks, etc. to prop up the incline

level

Recommendations:

  1. Be sure that you place the motion detector on the upper end of the incline (see the diagram above).
  2. Start by leveling the incline. Depending on how you set it up, you might want to measure and record the height of what will be the upper end of the track. When you raise the end, you can measure the height from the same location, then subtract to find h.
  3. Set up the motion detector to display a graph (Graph icon) of velocity vs. time. You can use the statistics capability (statistics icon) of the software to calculate the slope of this graph, which, of course, is the acceleration of the cart.
  4. Open the sampling options (Sampling Options icon) dialog, and set the detector to stop automatically after 2 seconds or so.
  5. Let the cart start down the incline, then start () the detector.
  6. Remember that you can restrict the statistical analysis to the "good part" of the graph.
  7. If you can't get decent data, you'll need to make some motion detector adjustments.
  8. When you are ready to take acceleration data, open Graphical AnalysisTM and type your acceleration and height data directly into its data table. Construct a graph of acceleration vs. height.
  9. Be sure to take at least a few trials at each height so you can get a good idea of the uncertainty in the acceleration calculation.

Results:

If you have made several runs at each height, it might not be necessary to add error bars to the graph. Draw the best fit regression line, and get regression statistics for the acceleration vs. height graph, assuming it looks linear. You can calculate "g" from the slope of this line, right? Also, be sure to determine the uncertainty in your value of "g". What is the percent of difference between your calculated value and an "accepted value" of "g" (consult the Handbook of Physics & Chemistry)?

Conclusions:

So, what do you think? In particular,

[Help] [Lab Index]
BHS -> Staff -> Mr. Stanbrough -> AP Physics-> Kinematics-> this page
last update July 17, 2000 by JL Stanbrough